Optimize Portfolio using Efficient Frontier

Demanding market conditions have precipitated the need for efficient portfolio management techniques that can help investors preserve and grow their capital. With risk and expected returns at the forefront of investment decision-making, the efficient frontier has emerged as one of the most popular portfolio optimization tools.
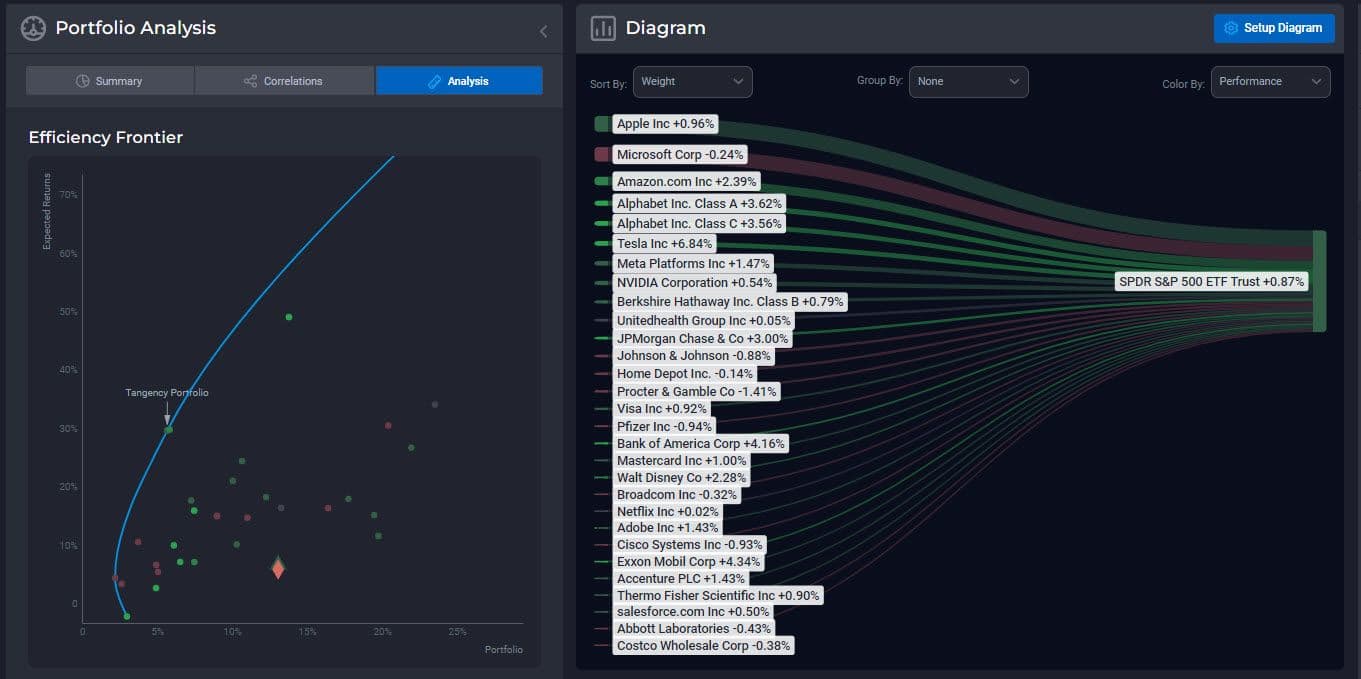
Drawing on a key tenet of the modern portfolio theory, the efficient frontier is a curve that represents the boundary between efficient and inefficient portfolios. It delineates the set of portfolios that offer the highest expected return for a given level of risk or the lowest level of risk for a given expected return. In other words, it is the efficient portfolio combination that provides the greatest return per unit of risk.
Since all investors have different risk tolerances, there is no single efficient frontier. Rather, each investor has their own efficient frontier that depends on their unique risk appetite. Establishing and adhering to an efficient frontier is essential for optimizing investment portfolios and achieving long-term goals but to use this framework effectively, it is important to first understand the concept and how it can be applied in practice.

Understanding the Efficient Frontier

First introduced in 1952 by Harry Markowitz, the efficient frontier is a tool that evaluates investment portfolios on a scale of risk and expected return. It does this by plotting efficient portfolios, which are a combination of assets with the highest expected return per unit of risk, on an efficient frontier curve. Based on this model, portfolios that lie on the efficient frontier are efficient, while those that skew below or above it are considered suboptimal due to their higher risk or lower expected return.
The efficient frontier framework is derived from the modern portfolio theory, which posits that it is possible to construct a diversified portfolio that will outperform any individual asset within it. This is because a portfolio of assets is not simply the sum of its parts but rather the result of the relationships and interactions between the assets. When these relationships are considered, it is possible to construct a portfolio that has less risk than any of the individual assets while still offering a higher expected return.
Returns are dependent on the expected return of each asset in the portfolio and the correlation between those assets. The higher the expected return and lower the correlation, the more efficient the portfolio. Risk, on the other hand, is measured by standard deviation, which captures the volatility of returns. Volatile assets will have a higher standard deviation and, therefore, a higher level of risk. Portfolios that fall on the right side of the efficient frontier have a higher expected return but are also riskier with those on the left side having lower risk and lower returns. The efficient frontier curve captures this relationship by plotting efficient portfolios along a continuum of risk and expected return - a visual representation of how divergent these two investment criteria can be.

Applying the Efficient Frontier to Portfolio Optimization

The efficient frontier is a powerful tool that can be used to optimize investment portfolios. By understanding where a portfolio falls on the efficient frontier, investors can make informed decisions about how to best allocate their assets. For example, if an investor is risk-averse and not willing to take on additional risk, they would want to choose a portfolio that falls on the left side of the efficient frontier curve. This would provide them with a lower level of risk but also a lower expected return.
On the other hand, an investor who is willing to take on additional risk in pursuit of higher returns would want to choose a portfolio that falls on the right side of the efficient frontier curve. This could potentially provide them with a higher return but also comes with the caveat of increased risk.
It is important to note that efficient frontier analysis is based on historical data and, as such, should be used as a guide rather than a prediction of future performance. Armed with this knowledge, investors can use this model to make more informed decisions about how to allocate and reallocate their assets to optimize their portfolios.

Key Assumptions and Considerations

There are a few key assumptions that efficient frontier analysis is based on, which investors should be aware of as they use this tool to optimize their portfolios.
The first is that efficient frontier analysis is based on the assumption of rational investors. This means that investors are risk-averse and, as such, will only take on additional risk if there is the potential for commensurate rewards. Efficient frontiers are constructed using a mean-variance optimization model, which relies on investors being able to accurately assess risk and expected returns. If efficient frontier analysis is used by investors who are not risk-averse or who cannot accurately assess risk and expected return, the results could be sub-optimal.
The second key assumption is that all assets are priced efficiently. This means that all assets are correctly priced in the market and there is no potential for arbitrage. While this assumption does not always hold true in the real world, it is again a useful assumption to make to model efficient portfolios.
The third key assumption is that all investors have access to the same information. This is critical as it ensures that all investors are making investment decisions based on the same information and no one has an advantage over the other. This assumption is sometimes referred to as the efficient markets hypothesis and is again useful for modeling efficient portfolios but may not hold true.
Finally, efficient frontier analysis is also limited by the amount of historical data available. With market conditions in a constant state of flux, efficient frontier analysis can only provide a snapshot in time of efficient portfolios. Investors should be aware of the potential for efficient frontier portfolios to underperform if there are unforeseen events that are not captured by historical data.
Despite these limitations, efficient frontier analysis is still a valuable tool that investors can leverage to optimize their portfolios. By understanding its key assumptions and limitations, investors can use this model to make more informed decisions about how to allocate their assets.

Calculating the Efficient Frontier

There are several ways to construct an efficient frontier but the most common is the capital market line (CML). The CML is derived from the Capital Asset Pricing Model (CAPM), which posits that the expected return of an asset is equal to the risk-free rate plus a premium for the asset's non-diversifiable risk. The CML is represented by the following equation:

ERp = Rf + SDp * (ERm – Rf) /SDm

where

ERp = Expected Return of the Portfolio

Rf = Risk-free Rate

SDp = Standard Deviation of Portfolio

ERm = Expected Return of the Market

SDm = Standard Deviation of Market

To construct the efficient frontier, investors need to calculate the expected return and standard deviation of each asset in their portfolio. This can be done using historical data or forecasts of future performance. Once the expected return and standard deviation of each asset is known, the efficient frontier can be graphed by plotting the expected return on the x-axis and standard deviation on the y-axis.
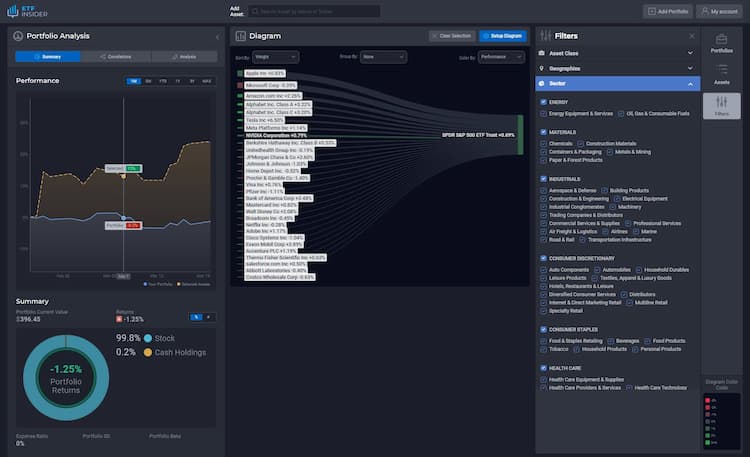
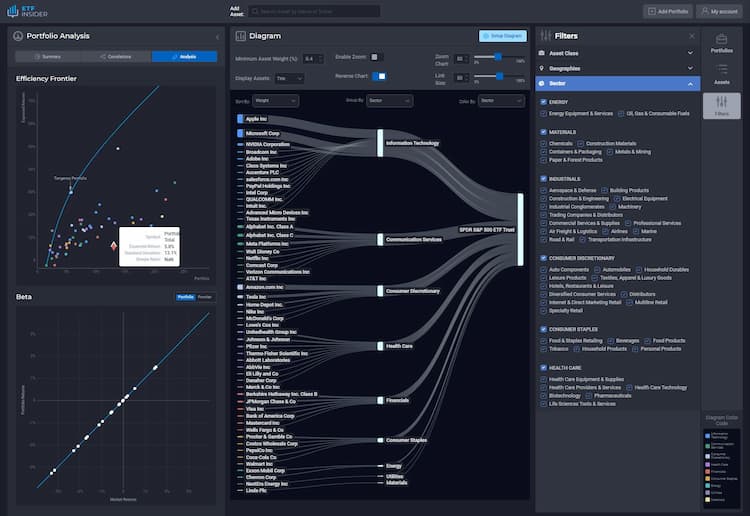
Due to the time and effort required to calculate the efficient frontier, many investors ignore this process and simply invest in a portfolio that is well diversified across asset classes, potentially leaving returns on the table. With ETFInsiders powerful efficiency frontier charting software, investors can quickly and easily see how efficient or inefficient their portfolio is without spending hours poring over data. Simply add assets to your virtual portfolio to quickly glean valuable insights that can assist you in making investment decisions that are aligned with your risk tolerance and investment objectives.